The Sierpiński triangle

Recently I’ve watched a video showing how to draw an approximation of the Sierpiński triangle on paper using the following steps¹:

Plot three points to form an equilateral triangle

Randomly select any point inside the triangle and consider that your current position

Randomly select any of the triangle’s vertices

Move half the distance from your current position to the selected vertex

Plot the current position

Go to step 3

I found it absolutely fascinating, and at the same time I was extremely skeptical. My mind couldn’t comprehend how following those simple steps could possibly yield this magnificent shape:


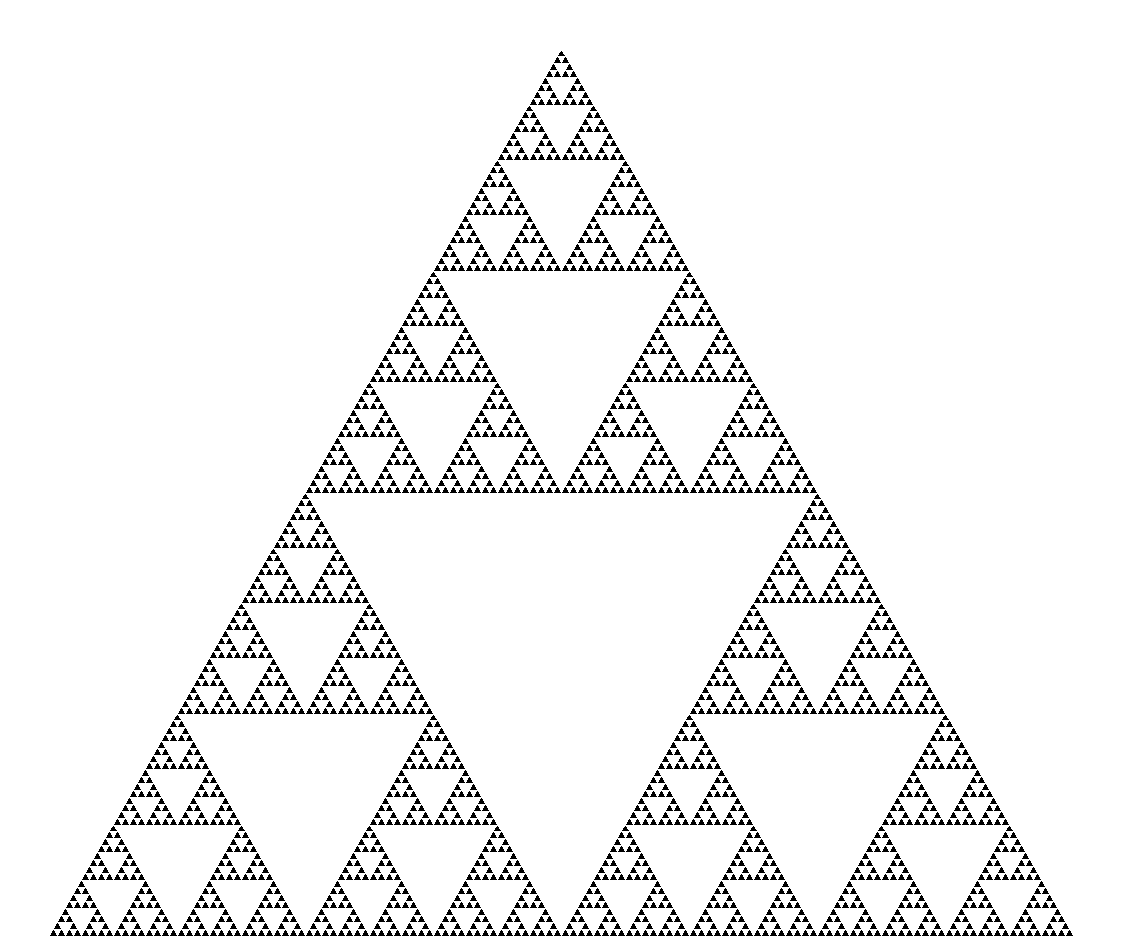
The Sierpiński triangle ²

Therefore, naturally, I had the overwhelming urge to write some code to verify the outcome of the video that I’d just watched.

So here goes:

<!-- index.html -->

<!DOCTYPE html>
<html>
  <head>
    <title>The Sierpiński triangle</title>
    <meta charset="UTF-8" />
  </head>

  <body style="background-color: black;">
    <canvas id="canvas" width="800" height="800"></canvas>
    <script src="index.js"></script>
  </body>
</html>

// index.js

(async function () {
  // Capture canvas element.
  const canvas = document.querySelector("#canvas");
  if (!canvas.getContext) {
    return;
  }

  // Capture canvas's 2D rendering context.
  const canvasContext = canvas.getContext("2d");
  const { width: canvasWidth, height: canvasHeight } = canvas;

  // Configure canvas's context.
  canvasContext.font = "30px monospace";
  canvasContext.fillStyle = "white";

  // Define the coordinates of the triangle's vertices.
  const lowerLeftVertex = { x: 0, y: canvasHeight };
  const lowerRightVertex = { x: canvasWidth, y: canvasHeight };
  const topVertex = { x: canvasWidth / 2, y: 0 };

  const vertices = [lowerLeftVertex, lowerRightVertex, topVertex];
  const midpoints = [];

  // Plot the triangle's vertices.
  vertices.forEach(drawPoint(canvasContext));

  // Repeat 20 thousand times.
  for (let i = 0; i < 20_000; i++) {
    // Select a vertex at random.
    const randomVertex = getRandomElement(vertices);
    // Get the last midpoint.
    const lastMidpoint =
      midpoints[midpoints.length - 1] ?? getRandomElement(vertices);

    // Compute the midpoint between the randomly selected vertex and the last
    // midpoint from the previous iteration (i.e. current position).
    const newMidpoint = computeMidpoint(randomVertex, lastMidpoint);
    midpoints.push(newMidpoint);

    // Draw the newly computed midpoint.
    drawPoint(canvasContext)(newMidpoint);
    // Re-render iteration count.
    updateIterationCount(canvasContext)(i);

    // Sleep for 1 millisecond to plot the points incrementally and not all at
    // once.
    await sleep(1);
  }
})();

function drawPoint(canvasContext) {
  return function (coordinates, size = 1) {
    const { x, y } = coordinates;

    canvasContext.beginPath();
    canvasContext.arc(x, y, size, 0, Math.PI * 2, true);
    canvasContext.fill();
  };
}

function updateIterationCount(canvasContext) {
  return function (i) {
    canvasContext.clearRect(0, 0, 200, 50);
    canvasContext.fillText(i, 0, 30);
  };
}

function getRandomElement(list) {
  const randomIndex = Math.floor(Math.random() * list.length);
  return list[randomIndex];
}

function computeMidpoint(firstPoint, secondPoint) {
  return {
    x: Math.floor(Math.abs((firstPoint.x + secondPoint.x) / 2)),
    y: Math.floor(Math.abs((firstPoint.y + secondPoint.y) / 2)),
  };
}

function sleep(millis) {
  return new Promise(function (resolve) {
    setTimeout(resolve, millis);
  });
}

And sure enough, it produces the promised result:

GIF showing the Sierpinski triangle being plotted